This module reviews Linear and Nonlinear Buckling Analysis
Background
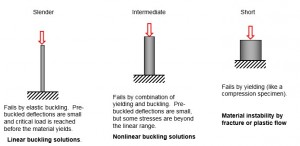
Buckling occurs as an instability when a structure can no longer support the existing compressive load levels. Many structural components are sufficiently stiff that they will never suffer any form of instability. In the figure these structures are classically described as ‘short’. In practice it is the relationship between radius of gyration and length that is the deciding factor and hence long span girders of heavy section could easily be clear of any instability mode. This type of structure would only fail in compression by local yielding if load levels can reach that extreme.
At the other extreme structures which are ‘slender’ could fail at load levels well below that required to cause compressive yielding. The failing mode tends towards the classic Euler buckling mode. For long thin rods and struts the Euler buckling calculation can be quite accurate. Buckling here is of a bifurcation type – there is a rapid transition from axial loading response to a lateral response which is usually catastrophic.
A very large number of structures fall into the intermediate category where the Euler buckling calculation is not very accurate and can tend to seriously overestimate the critical buckling load. The transition to instability is more gradual in this category. The structure is able to carry increasing loads, with perhaps changes in deformed shape and plasticity, until a maximum or limit load is reached. At this point instability occurs. This may be catastrophic, or the structure may transition to a new mode shape which can carry further load. Examples include the initial buckling of a drink can, initial buckling of a thin wing spar shear web or the light frame of a screen door.